
Rank Nullity Theorem Example 2 Linear Algebra Griti YouTube
Rank-Nullity Theorem Let and be vector spaces over a field , and let be a linear transformation . Assuming the dimension of is finite, then where is the dimension of , is the kernel, and is the image . Note that is called the nullity of and is called the rank of . See also Kernel, Null Space, Nullity, Rank This entry contributed by Rahmi Jackson
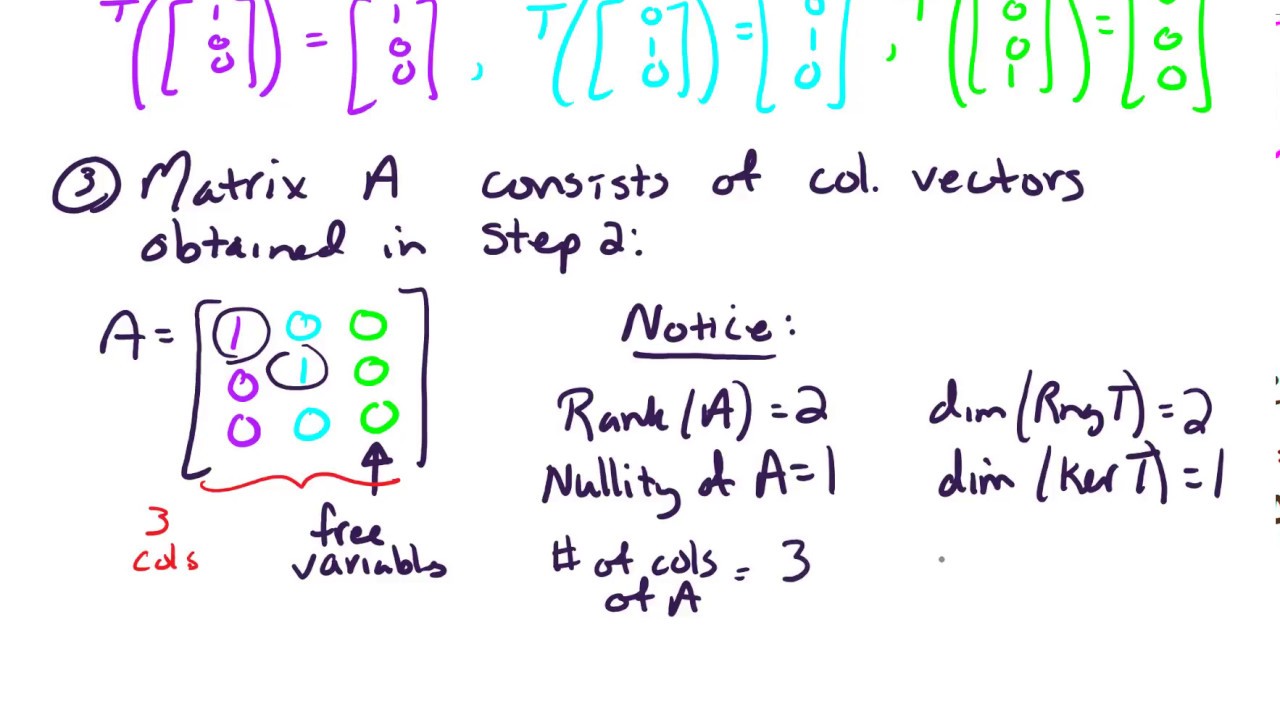
Sec 4.8 Example 1 Kernel, range, and ranknullity for a linear transformation YouTube
The Rank-Nullity Theorem helps here! Linear Algebra Dimension, Rank, Nullity Chapter 4, Sections 5 & 6 9 / 11. Example Suppose A is a 20 17 matrix. What can we say about A~x = ~b? Recall that NS(A) is a subspace of R17 and CS(A) is a subspace of R20.
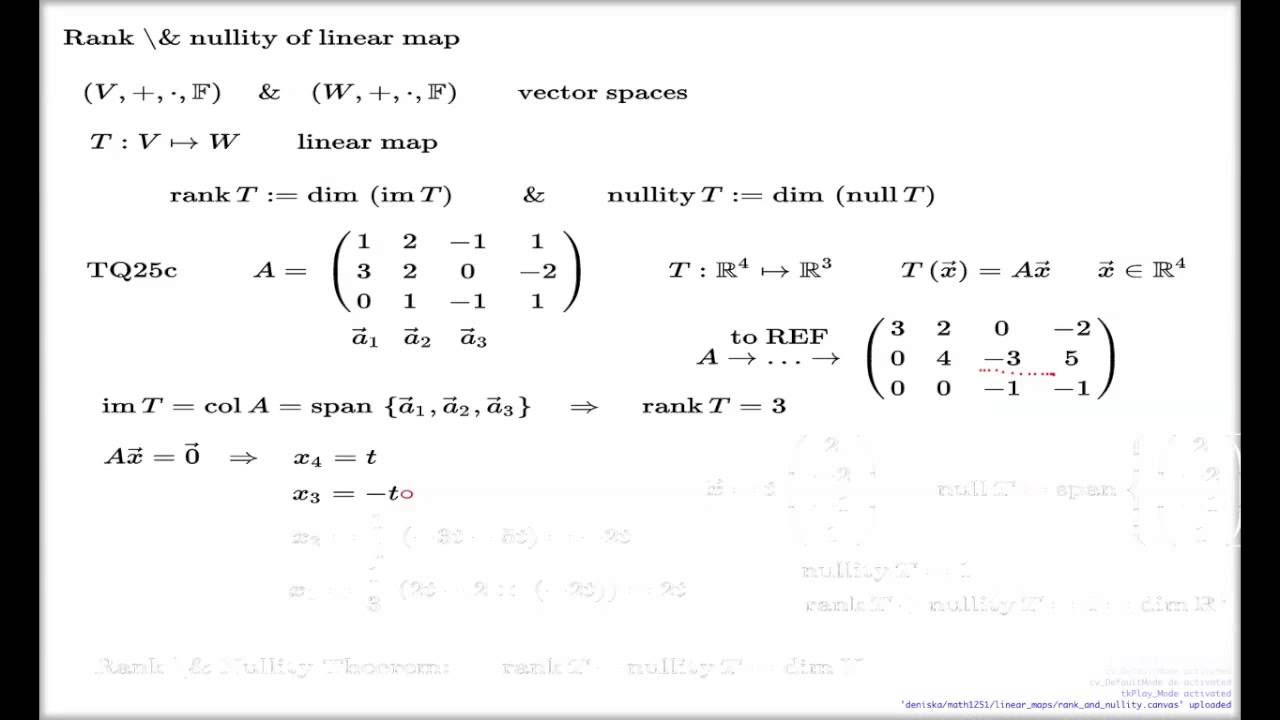
Rank and nullity of linear map, Rank and Nullity Theorem YouTube
Rank-Nullity Theorem Homogeneous linear systems Nonhomogeneous linear systems The Rank-Nullity Theorem De nition When A is an m n matrix, recall that the null space of A is nullspace(A) = fx 2Rn: Ax = 0g: Its dimension is referred to as the nullity of A. Theorem (Rank-Nullity Theorem) For any m n matrix A, rank(A)+nullity(A) = n:
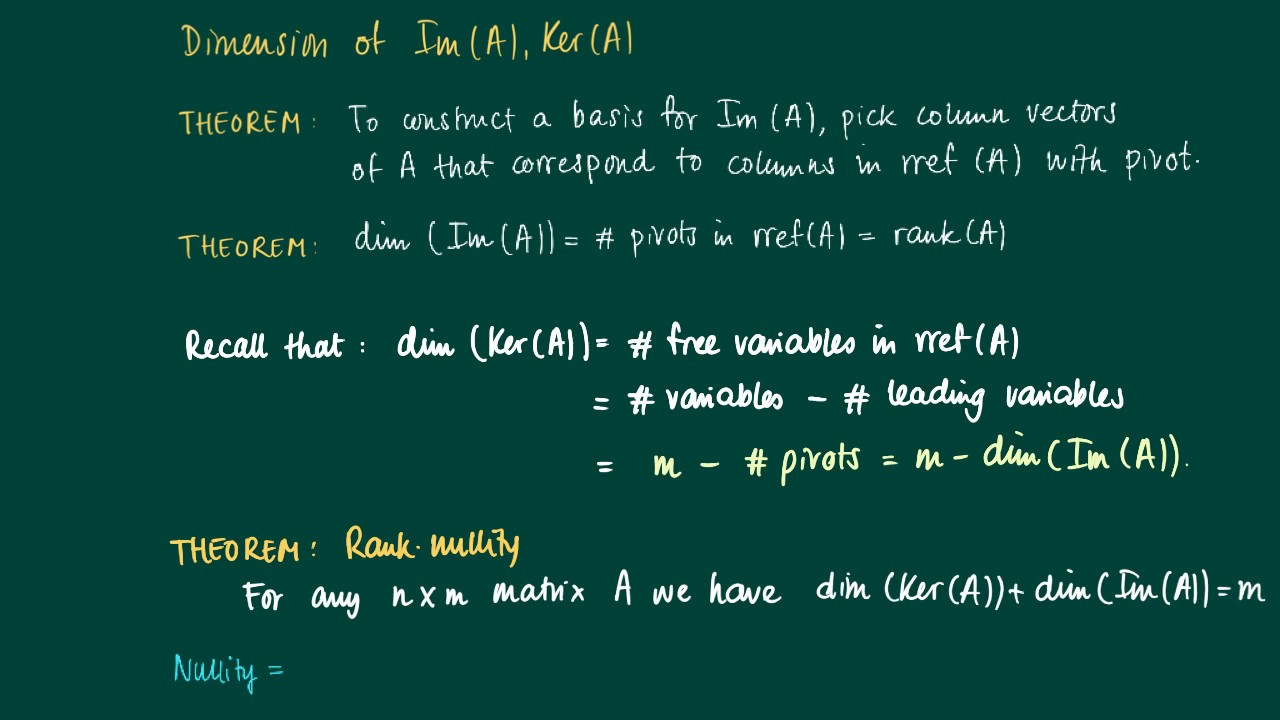
Math 2.9.8. Rank nullity theorem YouTube
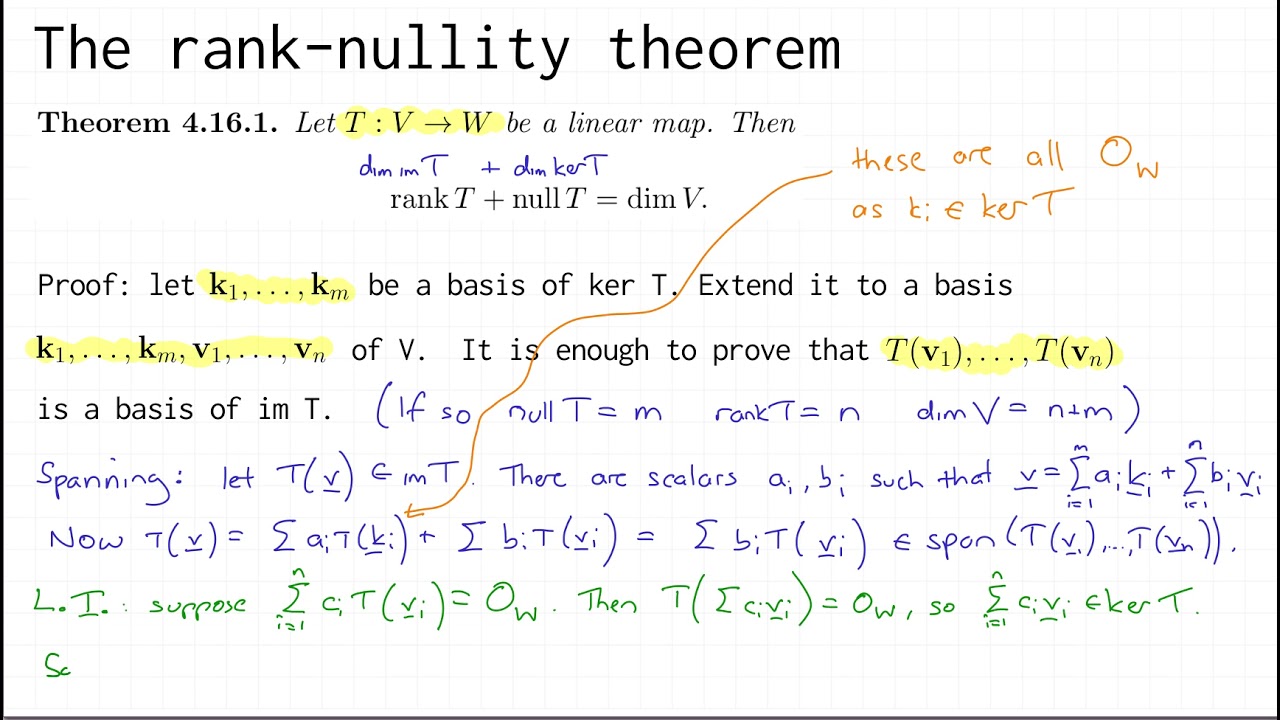
The rank of T, written rank T is dim im T. Example 4.16.1 . Returning to the differentiation example from the end of the last lecture, D : ℝ ⩽ n [ x ] → ℝ ⩽ n [ x ] has nullity 1 (since its kernel was one-dimensional, spanned by the constant polynomial 1) and rank n , since its image had a basis 1 , x ,. , x n − 1.

Linear Algebra Lecture 39 Rank Nullity Theorem for Linear Transformations YouTube
The Rank-Nullity Theorem Definition 1. The dimension of a non-zero subspace, H, denoted dim H, is the number of vectors in any basis for H. The dimension of the zero subspace f0g is zero. Definition 2. The rank of a matrix, A 2 Rm n, denoted rank A, is the dimension of the column space of A, rank A = dim (Col(A)) :
Linear Algebra Part 35 RankNullity Theorem YouTube
Rank Theorem. rank ( A )+ nullity ( A )= n . (dimofcolumnspan) + (dimofsolutionset) = (numberofvariables). The rank theorem theorem is really the culmination of this chapter, as it gives a strong relationship between the null space of a matrix (the solution set of Ax = 0 ) with the column space (the set of vectors b making Ax = b consistent.

The RankNullity Theorem (part 1) YouTube
Example 2.9. 1: Rank and nullity. Here is a concrete example of the rank theorem and the interplay between the degrees of freedom we have in choosing x and b in a matrix equation A x = b. Consider the matrices. A = ( 1 0 0 0 1 0 0 0 0) and B = ( 0 0 0 0 0 0 0 0 1). If we multiply a vector ( x, y, z) in R 3 by A and B we obtain the vectors A x.

Rank nullity theorem for matrices YouTube
Given a linear transformation L: V → W, we want to know if it has an inverse, i.e., is there a linear transformation M: W → V such that for any vector v ∈ V, we have MLv = v, and for any vector w ∈ W, we have LMw = w. A linear transformation is just a special kind of function from one vector space to another.
Rank nullity theorem YouTube
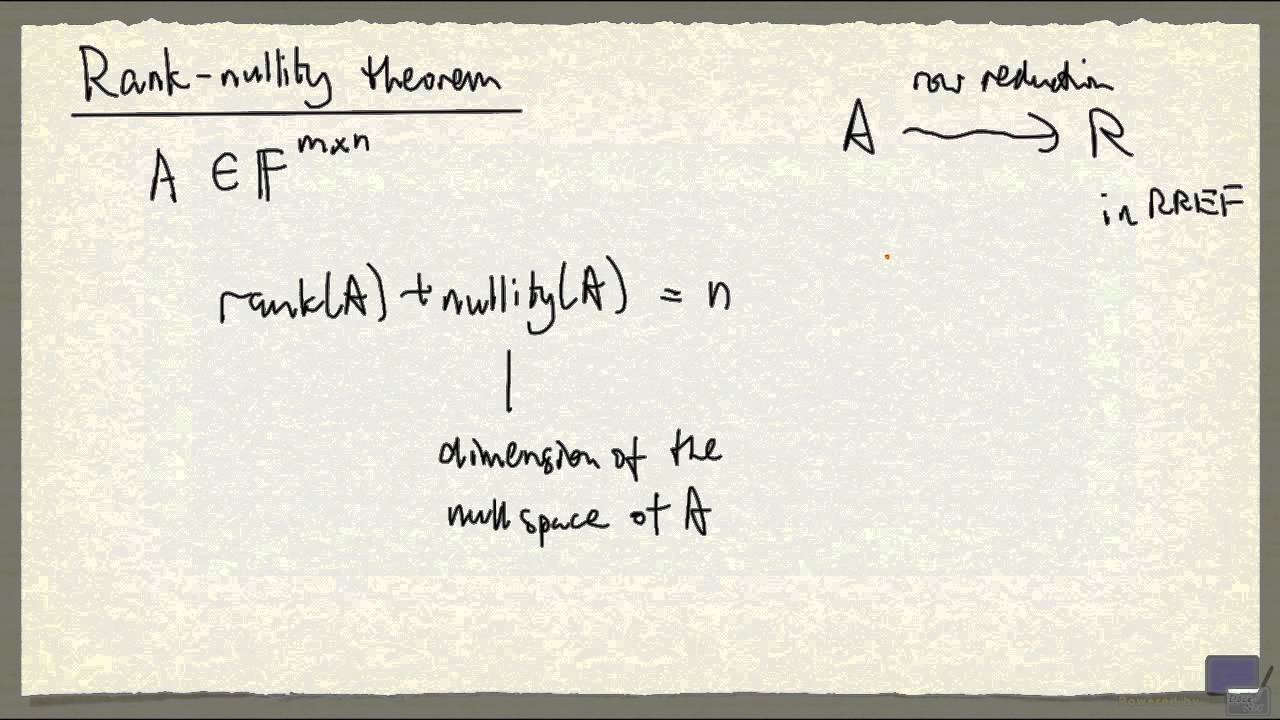
The rank nullity theorem September 17, 2007 Let A be an n×m matrix. We would like to use Gauss elimination to find a basis for the column space of A. In order to do this we need to understand more about the relationship between A and its reduced row echelon form.

Ranknullity theorem Lecture notes 22 Applied Mathematics StuDocu
Rank Theorem. rank ( A )+ nullity ( A )= n . (dimofcolumnspan) + (dimofsolutionset) = (numberofvariables). The rank theorem theorem is really the culmination of this chapter, as it gives a strong relationship between the null space of a matrix (the solution set of Ax = 0 ) with the column space (the set of vectors b making Ax = b consistent.

Rank Theorem (RankNullity Thm), 4 Fundamental Subspaces, Invertible Matrix Theorem, Change of
4.9 The Rank-Nullity Theorem In Section 4.3, we defined the null space of a real m n matrix × A to be the set of all real solutions to the associated homogeneous linear system Ax 0. Thus, = nullspace(A) {x n = ∈ R Ax : 0 = }. The dimension of nullspace(A) is referred to as the nullity of A and is denoted nullity(A).

rank of linear transformation rank nullity Theorem Matrix Representation BHU 2018 linear algebra
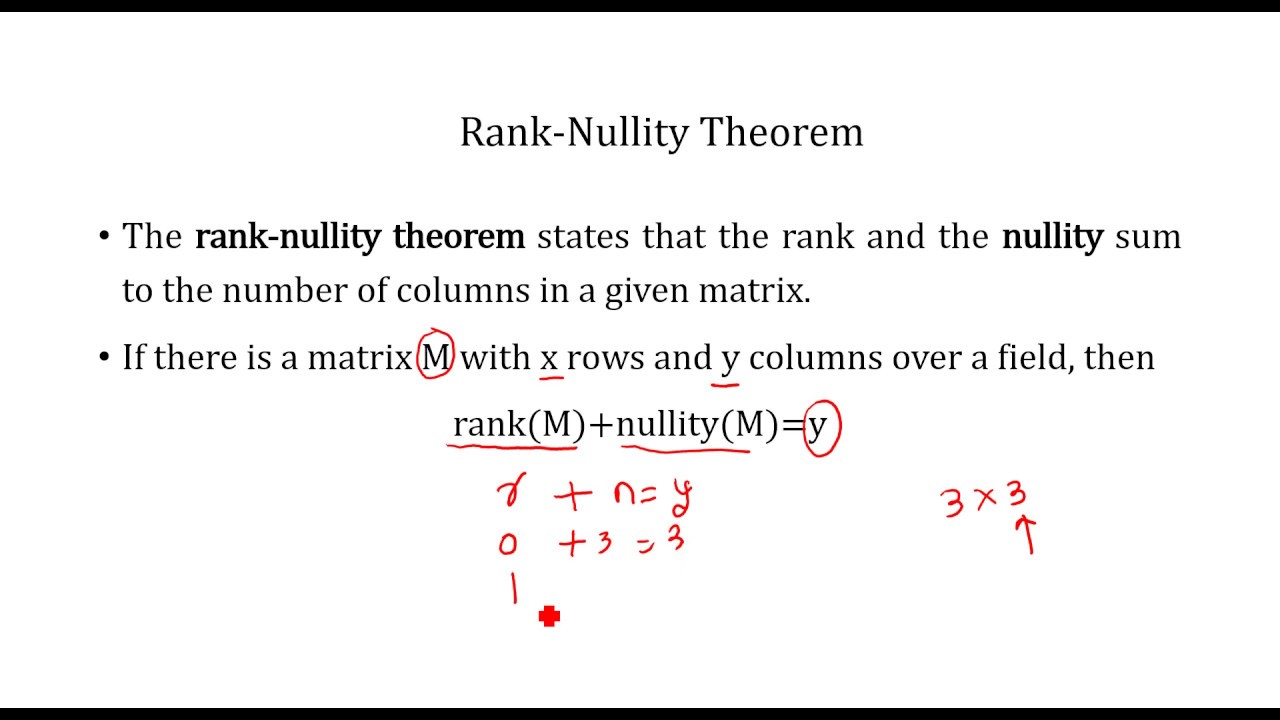
Rank-nullity theorem The nullity (dimension of the nullspace) and the rank (dimension of the range) of a matrix add up to the column dimension of , . Proof: Let be the dimension of the nullspace ( ). Let be a matrix such that its columns form an orthonormal basis of . In particular, we have .

MATH0005 L59 the ranknullity theorem YouTube
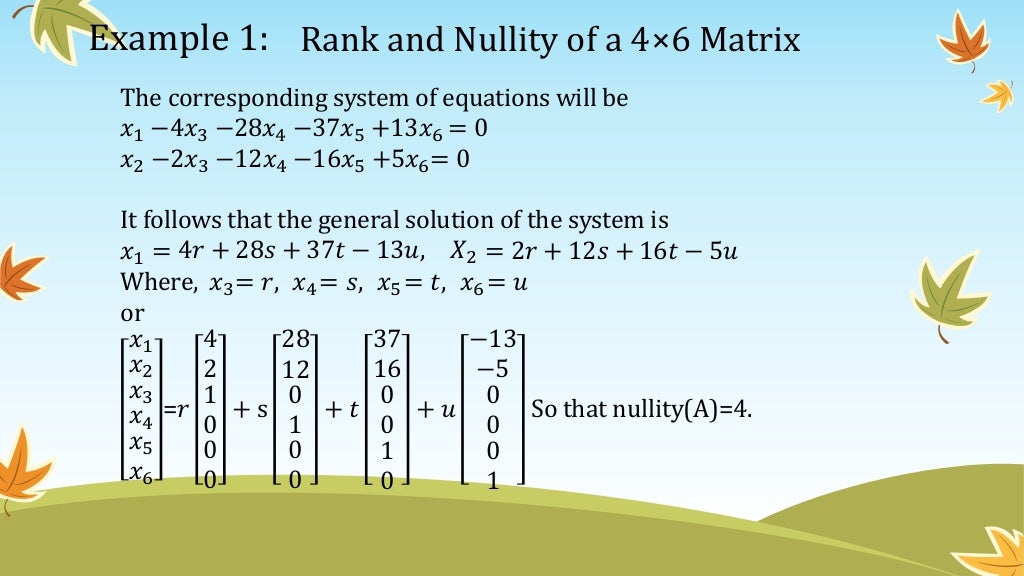
The Rank of a Matrix is the Dimension of the Image Rank-Nullity Theorem Since the total number of variables is the sum of the number of leading ones and the number of free variables we conclude: Theorem 7. Let M be an n m matrix, so M gives a linear map M : Rm!Rn: Then m = dim(im(M)) + dim(ker(M)): This is called the rank-nullity theorem.
Rank nullity theorem
University of Oxford mathematician Dr Tom Crawford introduces the concepts of rank and nullity for a linear transformation, before going through a full step-.
4.16 the ranknullity theorem YouTube
The rank-nullity theorem is a theorem in linear algebra, which asserts: the number of columns of a matrix M is the sum of the rank of M and the nullity of M; and the dimension of the domain of a linear transformation f is the sum of the rank of f (the dimension of the image of f) and the nullity of f (the dimension of the kernel of f ).
Week 9 Ranknullity theorem YouTube
Rank is the number of leading column or non-zero row vectors of row-reduced echelon form of the given matrix, and the number of zero columns is the nullity. The nullity of a matrix is the dimension of the null space of A, also called the kernel of A. If A is an invertible matrix, then null space (A) = {0}.